Minisymposium ''Perspectives of Gradient-Robustness''
Berlin, July 08, 2021
Alexander Linke introduced the concept of pressure/gradient robustness of finite
element discretizations for flow problems, which allows for a significant
improvement of the physical quality and efficiency of numerical simulations
by reducing errors from non-trivial gradient fields in the momentum balance.
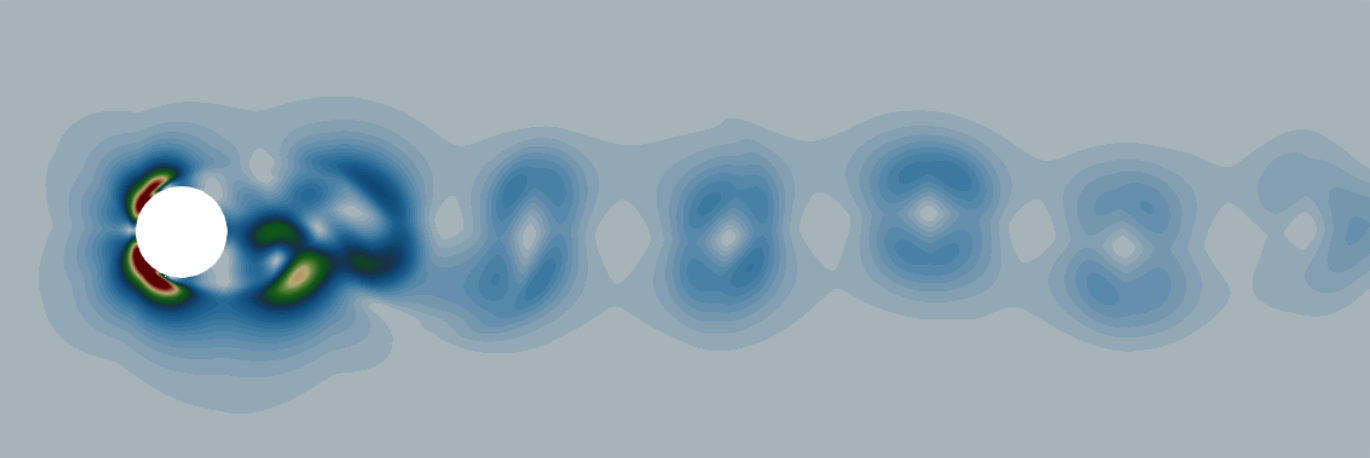
The picture (taken from Figure 9.3. in
this
publication (exterior link to pdf)) shows a snap-shot of such a non-trivial
gradient field from the material derivative in a periodic Kármán vortex street.
On the occasion of Alexander Linke's retirement as active scientist we
want to celebrate his achievements and discuss the perspectives of the
concept of gradient robustness from a theoretical and applied point of
view.
Organizers WIAS Berlin
Jürgen Fuhrmann | Volker John | Christian MerdonPreliminary Program
| 13:00 -- 13:45 | J. Schöberl (Technische Universität Wien) Software tools for robust finite element methods (abstract)
In recent years many advanced finite element methods with improved robustness properties have been proposed. We are thinking of mixed and discontinuous Galerkin methods, with partial continuity of vector fields, and facet variables for hybridization.
|
| 13:45-- 14:30 | Th. Apel/Volker Kempf (Universität der Bundeswehr
München) A pressure-robust discretization of the Stokes problem on anisotropic meshes (abstract)
Anisotropic finite element meshes are particularly efficient when the
solution of the problem has anisotropic features like boundary layers
or edge singularities. The Crouzeix-Raviart method is known to be
inf-sup stable on anisotropic meshes, yet not pressure-robust.
Inf-sup stable finite element schemes with discontinuous pressure can
be made pressure-robust by a modified discretization of the exterior
forcing term using H(div)-conforming reconstruction operators like the
Raviart-Thomas or Brezzi-Douglas-Marini interpolants.
|
| 14:30 -- 15:00 | Coffee Break |
| 15:00 -- 15:45 | M. Neilan (University of Pittsburgh) Quasi-optimal error estimates of pressure-robust schemes for the Stokes problem (abstract)This talk discusses error estimates of the pressure-robust schemes for the Stokes problem introduced by A. Linke and collaborators. These methods introduce a nonstandard discretization of the right-hand side function, leading to consistency errors. We discuss various approaches and mathematical tools to analyze the methods with a focus on low-regularity solutions. This is joint work with Alexander Linke, Christian Merdon, and Felix Neumann. |
| 15:45 -- 16:30 | Ch. Merdon (WIAS Berlin) On the importance of gradient-robustness in convection stabilisation and compressible flows (abstract)
In high Reynolds number flows there are two possible sources of discretisation errors: a lack of gradient-robustness and dominant convection. The latter one persists if divergence-free discretisations like the Scott-Vogelius element are used and requires some kind of convection stabilisation. However, the gains from gradient-robustness can be compromised if a convection stablisation is used that is not gradient-robust like the SUPG convection stabilisation. The talk presents a novel residual-based least-square convection stabilisation which is based on the vorticity equation. This stabilisation preserves the gradient-robustness of the original method and so allows pressure-independent velocity estimates and the best known rates for the $L^2$-norm in the linearised Oseen case [1].
|