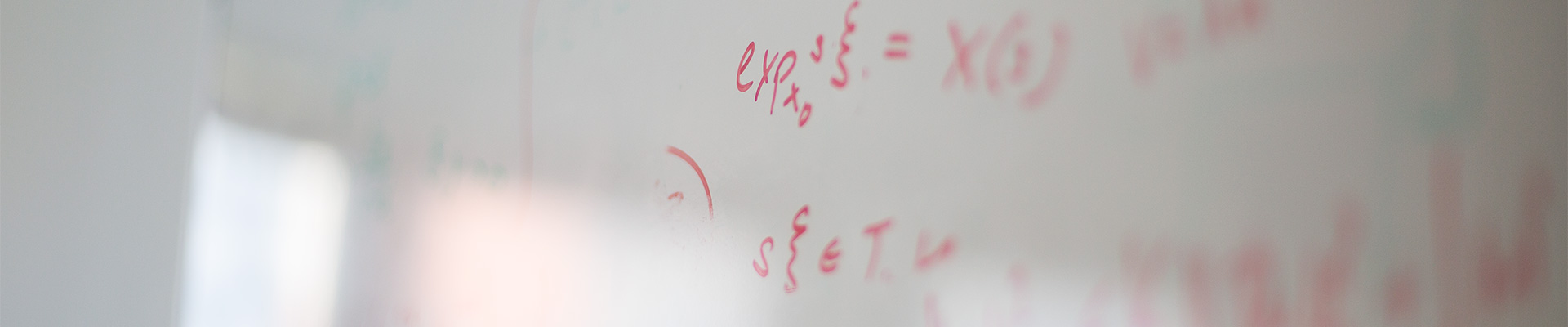Conditional excursion representation for a class of interacting superprocesses
Authors
- Li, Zenhu
- Wang, Hao
- Xiong, Jie
2010 Mathematics Subject Classification
- 60J80 60G57 60J35
Keywords
- superprocess, interaction, immigration, non-linear SPDE, conditional log-Laplace functional, excursion law
DOI
Abstract
A class of interacting superprocesses, called superprocesses with dependent spatial motion (SDSMs), has been introduced and characterized in Wang citeWang98 and Dawson et al. citeDLW01. In this paper, we give a construction or an excursion representation of the non-degenerate SDSM with immigration by making use of a Poisson system associated with the conditional excursion laws of the SDSM. As pointed out in Wang citeWang98, the multiplicative property or summable property is lost for SDSMs and immigration SDSMs. However, summable property is the foundation of excursion representation. This raises a sequence of technical difficulties. The main tool we used is the conditional log-Laplace functional technique that gives the conditional summability, the conditional excursion law, and the Poisson point process for the construction of the immigration SDSMs.
Download Documents