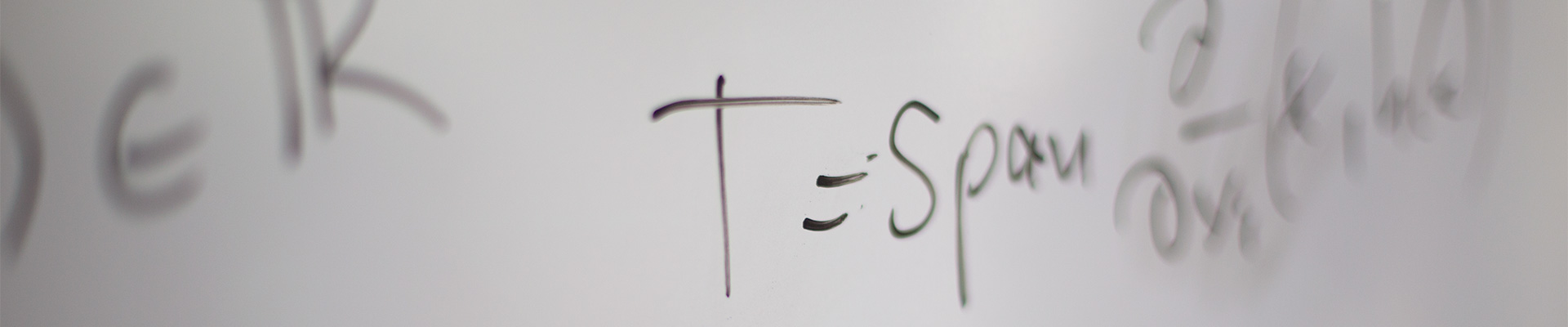Neutral delay differential equation Kerr cavity model
Authors
- Vladimirov, Andrei G.
ORCID: 0000-0002-7540-8380 - Dolinina, Daria
ORCID: 0009-0000-6829-7806
2020 Mathematics Subject Classification
- 78A60 34K40
2010 Physics and Astronomy Classification Scheme
- 42.65.-k, 42.65.Sf, 42.65.Pc, 42.65.Tg.
Keywords
- Temporal cavity solitons, neutral delay differential equation model, passive optical cavity
DOI
Abstract
A neutral delay differential equation (NDDE) model of a Kerr cavity with external coherent injection is developed that can be considered as a generalization of the Ikeda map with second and higher order dispersions being taken into account. It is shown that this model has solutions in the form of dissipative solitons both in the limit, where the model can be reduced to the Lugiato--Lefever equation (LLE), and beyond this limit, where the soliton is eventually destroyed by the Cherenkov radiation. Unlike the standard LLE the NDDE model is able to describe the overlap of multiple resonances associated with different cavity modes.
Appeared in
- , 109 (2024), pp. 024206/1--024206/10, DOI 10.1103/PhysRevE.109.024206 .
Download Documents