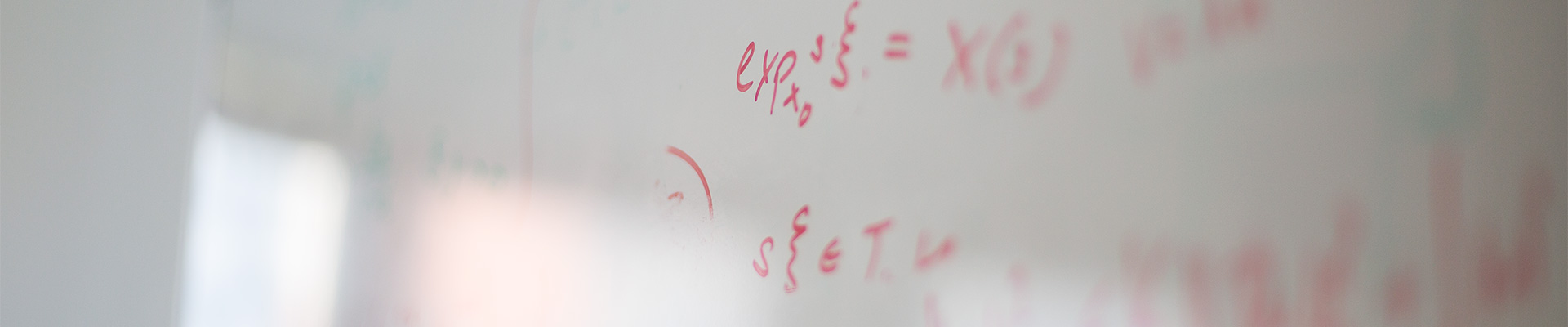Properties of the steady state distribution of electrons in semiconductors
Authors
- Muscato, Orazio
- Wagner, Wolfgang
- Di Stefano, Vincenza
2010 Mathematics Subject Classification
- 82D37 65C05
Keywords
- Boltzmann-Poisson equation, semiconductors, electron transport, steady state distribution, Monte Carlo algorithm
DOI
Abstract
This paper studies a Boltzmann transport equation with several electron-phonon scattering mechanisms, which describes the charge transport in semiconductors. The electric field is coupled to the electron distribution function via Poisson's equation. Both the parabolic and the quasi-parabolic band approximations are considered. The steady state behaviour of the electron distribution function is investigated by a Monte Carlo algorithm. More precisely, several nonlinear functionals of the solution are calculated that quantify the deviation of the steady state from a Maxwellian distribution with respect to the wave-vector. On the one hand, the numerical results illustrate known theoretical statements about the steady state and indicate possible directions for future studies. On the other hand, the nonlinear functionals provide tools that can be used in the framework of Monte Carlo algorithms for detecting regions in which the steady state distribution has a relatively simple structure, thus providing a basis for domain decomposition methods.
Appeared in
- Kinet. Relat. Models, 4 (2011) pp. 809--829.
Download Documents