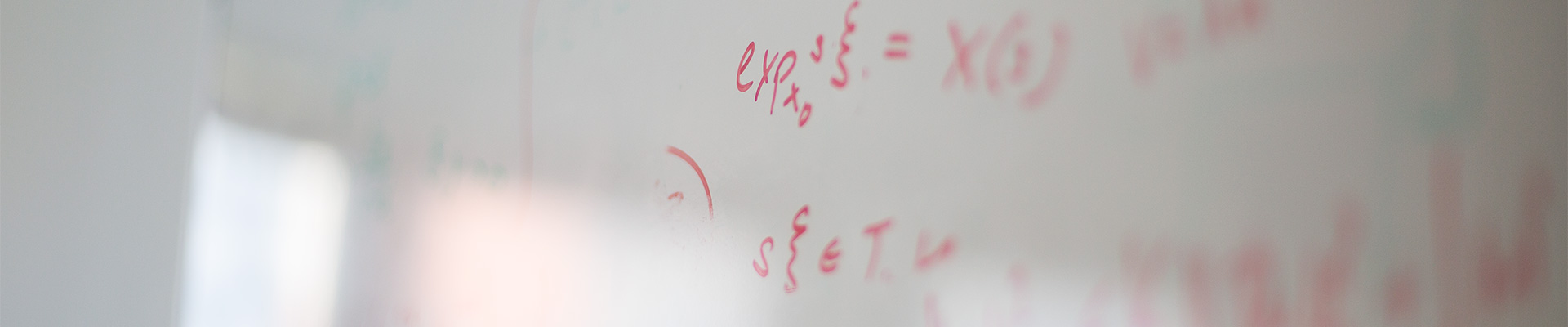Boundary element discretization of Poincaré-Steklov operators.
Authors
- Schmidt, Gunther
2010 Mathematics Subject Classification
- 65N38 65N30 65N55 35J25
Keywords
- Galerkin method, Poincaré-Steklov operators, boundary element method, domain decomposition methods, finite element
DOI
Abstract
This paper is devoted to the construction of a discretization of Poincaré-Steklov (PS) operators for elliptic boundary value problems with the boundary element method (BEM). PS operators are natural mathematical tools for the investigation of boundary value problems and their numerical solution with domain decomposition (DD) methods based on the finite element (FE) solution of the subproblems (cf. [1], [9]). We will show that the discretizations of PS operators with a direct Galerkin BEM possess the same properties as the FE discretizations if the boundary elements satisfy some natural conditions. Hence the given construction provides a base for the analysis of different DD methods using the BE solution of subproblems, of the coupling of FE and BE methods and related problems.
Appeared in
- Numer. Math., 69 (1994), pp. 83--101
Download Documents