Optimal control of surface heat treatments

[Next]:

Hysteresis phenomena

[Up]:

Project descriptions

[Previous]:

Stationary solutions of two-dimensional heterogeneous energy

[Contents]

[Index]

Optimal control of surface heat treatments

Collaborator: D. Hömberg , Th. Jurke , W. Weiss

Cooperation with: LASERVORM Volumen- und Oberflächenbearbeitung (Mittweida), pro-beam HÖRMANN GmbH (Neukirchen), J. Soko $\l$ owski (Université de Nancy I, France), H.-J. Spies (TU Bergakademie Freiberg), S. Volkwein (Karl-Franzens-Universität Graz, Austria)

Supported by: Stiftung Industrieforschung, Köln

Description:

In most structural components in mechanical engineering, there are surface parts which are particularly stressed. The aim of surface hardening is to increase the hardness of the corresponding boundary layers by rapid heating and subsequent quenching. This heat treatment leads to a change in the microstructure, which produces the desired hardening effect.

Depending on the respective heat source one can distinguish between different surface hardening procedures, the most important ones being induction hardening and radiation treatments like laser- and electron-beam hardening.

Fig. 1: Laser surface hardening of the steel X35CrMo17: Comparison of simulation (right) with experiment (left)
$\makeatletter \@ZweiProjektbilderNocap[h]{0.4\linewidth}{hoemberg_fig1_02.ps}{hoemberg_fig2_02.ps} \makeatother$

Fig. 2: Electron beam surface hardening of the steel C45: Comparison of simulation (right) with experiment (left)
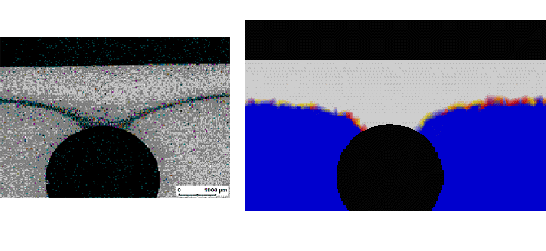
$\ProjektEPSbildNocap {12.51cm}{hoemberg_fig3-4.ps} \begin{imagesonly} \addtocounter{projektbild}{-1}\end{imagesonly}$

To complete our industrial project on simulation and control of laser- and electron-beam hardening a Mathematica tool has been developed and used to identify the transformation parameters of our phase transition model for a number of relevant steels. These parameters have been added to the data base of WIAS-SHarP. Numerical simulations for all these steels, with varying laser intensity and different workpiece geometries, have been carried out. The results, which are in good agreement with experiments (cf. Figures 1, 2), are documented in [1].

Another important control problem related to surface hardening is the optimal design of inductor coils for induction surface hardening. In [4] the speed method has been applied to investigate the sensitivity of solutions to the state equations with respect to perturbations of the inductor. In the case of inductors constructed from space curves it has been shown in [2] that one can relate the resulting shape gradient to a perturbation of the generating curve.

Fig. 3: Phase transitions with thermomechanics -- interdependence of physical quantities
$\ProjektEPSbildNocap {0.8\textwidth}{hoemberg_fig5_02.eps} \begin{imagesonly} \addtocounter{projektbild}{-1}\end{imagesonly}$

Figure 3 shows the complex interdependence of the relevant physical quantities for phase transitions in steel. The interplay between temperature $\theta$ and volume fraction z is well understood (cf., e.g., [1]). The metallurgical phases z_i have material parameters with different thermal characteristics, hence their effectice values have to be computed by a mixture ansatz. The different densities of the metallurgical phases result in a different thermal expansion. This thermal and transformation strain is the major contribution to the evolution of internal stresses during heat treatments. Experiments with phase transformations under applied loading show an additional irreversible deformation even when the equivalent stress corresponding to the load is far below the normal yield stress. This effect is called transformation-induced plasticity. The irreversible deformation leads to a mechanical dissipation that acts as a source term in the energy balance.

Neglecting the influence of internal stresses on the transformation kinetics, a consistent mathematical model which takes care of all these effects has been developed and analyzed in [2], [3].

References:

A. BUCHWALDER, D. HÖMBERG, TH. JURKE, H.-J. SPIES, W. WEISS, Simulation der Strahlhärtung von Stahl mit WIAS-SHarP, Tech. Rep. no. 3, WIAS, 2002.
D. HÖMBERG, Induction heat treatments -- Modeling, analysis and optimal design of inductors, habilitation thesis, Technische Universität Berlin, 2002.
$\dito$ , A mathematical model for induction hardening including mechanical effects, WIAS Preprint no. 766, 2002.
D. HÖMBERG, J. Soko $\l$ owski, Sensitivity analysis of an eddy current problem related to induction heating, WIAS Preprint no. 776, 2002.